Summary
Zero-one (0-1) integer linear programming represents a mathematical method that uses a set of binary answers (yeas and no) to solve with two mutually exclusive options (Anderson et al., 2016). The method has a wide application in different areas of life, ranging from the world of finance to everyday problems that people face regularly. In zero-integer problems, every variable is represented solely by either 0 or 1 and is concerned with either selecting or rejecting a specific option that would lead to an ultimate conclusion.
The research article by Gholamnejad (2008) should be mentioned as it is concerned with the application of the zero-one integer programming in real life, within the context of pit mining sequences. Even though the article is quite dated, it is useful to further one’s understanding of how integer programming can be used. The objectives of using the mode, according to the researcher, were concerned with the proper utilization of equipment at mining facilities ensuring the efficiency of ore production, reaching minimum degrees of deviation from both long- and medium-term plans, as well as ensuring that maximal flexibility of systems is being achieved.
When developing a model to use during pit mining, the researcher suggested using several factors. For instance, it is necessary to account for the characteristics of waste and ore blocks that are exposed to mining, the capacities of equipment that is used in mining, long-term and medium-term plans of production, as well as the overall state of systems as a whole. When applying the zero-one integer problem, the scholar reached several conclusions.
First, it is essential to note that the model provided more complexities for the processes at short-term mines. Despite this issue, such a kind of modeling ensures that each block in the block modeling has a “free face for loading and transportation equipment” (Gholamnejad, 2008, p. 762). Moreover, the model ensures that each period of handling resources has predetermined standards of quality when preparing for further stages of processing.
Reaction
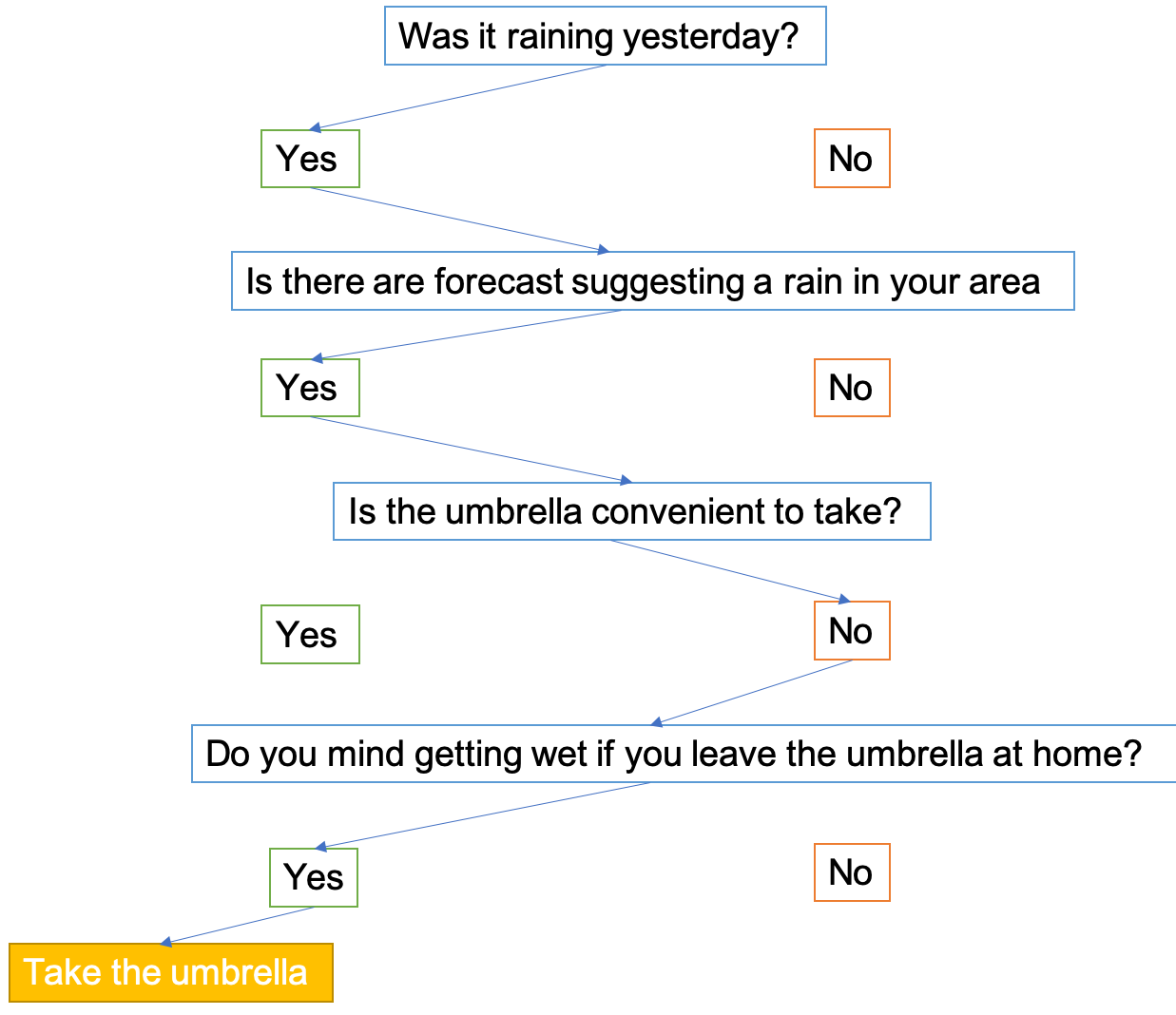
In the article, the author does not discuss how one can apply the simple zero-one integer model in situations that do not involve mining. Therefore, it is imperative to develop a model by oneself to understand its application in real life. For instance, when deciding whether or not to take an umbrella one day, a series of yes or no questions should be answered to come to one of two mutually exclusive options.
The questions to answer with yes/no options should include: was it raining yesterday? Is there are a forecast suggesting rain in your area? Is the umbrella convenient to take? Do you mind getting wet if you leave the umbrella at home? The scheme below represents the decision-making process within the model and will lead to deciding whether or not an umbrella should be taken. Overall, the article by Gholamnejad (2008) is limited by focusing only on the mining industry, failing to provide examples for the real-life use of the model. In this reaction, it was attempted to offer a simple breakdown of how the model can be used for real-life situations, without complicating things. It is also important to note that more studies should be conducted explaining the use of the model.
References
Anderson, D. R., Sweeney, D. J., Williams, T. A., Camm, J. D., Cochran, J. L., Fry, M. J., & Ohlmann, J. W. (2016). Quantitative methods for business with CengageNOW (13th ed.). Boston, MA: Cengage Learning.
Gholamnejad, J. (2008). A zero-one integer programming model for open pit mining sequences. The Journal of The Southern African Institute of Mining and Metallurgy, 108, 759-762.