Introduction
The expected return from individual securities carries some degree of risk. Risk is defined as the standard deviation from the expected return. More dispersion or variability from a security’s return means the security is riskier than one with less dispersion. In simple terms securities carry differing degrees of expected risk that may lead investors to have a notion of holding more than one security at a time, in an attempt to spread risks of the investment. These risks can be grouped as market risk and asset risk. The risk that affects the whole is usually cuts across the industries and does not affect one industry is market risk. While the risk that affects a certain asset is asset risk.Because market risks have market wide effects, they are sometimes called market risks (Fischer and Jordan, 2007).
The procedures used to arrive at the conclusion are laid out step by step. Although some assumptions are made before the final conclusion, but this financial analysis assumes the nature of real-time estimation of any investment which future yields matter a lot.
Combining the Pioneer and the market
Expected return
Holding A Pioneer stock and the market is less risky than holding Pioneer security, is it possible to reduce the risk of a portfolio by incorporating into it a security whose risk is greater than that of any of the investments held initially. Assuming the ratio of holding is 0.01% to 99.99%, then their expected return, risk premium, and standard deviation of the portfolio will be as shown below:
The portfolio consisting of 0.01% Pioneer and 99.99% the market and the average return of this portfolio can be thought of as the weighted average return of each security in the portfolio; that is:
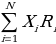
Where:
- Rp = expected return to portfolio
- Xi = proportion of total portfolio invested in security i
- Ri = expected return to security i
- N = total number of securities in portfolio
Therefore: Rp = (0.01%)(11%) + (99.99%)(12.5%) = 12.50%
Expected standard deviation
Diversification is meant to reduce risk and the key was not that two provided twice as much diversification as one, but that by investing in securities with negative or low covariance among themselves, we could reduce the risk. This was described by Markowitz’s in his efficient diversification theory. The theory states that to reduce risk stocks of less positive correlation should be combined. In general, the lower the correlation of securities in the portfolio, the less risky the portfolio will be. This is true regardless of how risky the stocks of the portfolio are when analysed in isolation. It is not enough to invest in many securities, it is necessary to have the right securities (Gordon, Jeffrey and Sharpe, 2000).
Then the standard deviation will be calculated as follows;
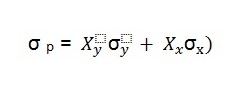
Where:
- σp = portfolio standard deviation
- Xx= percentage of total portfolio value in Pioneer
- Xy = percentage of total portfolio value in market
- σ x = standard deviation of pioneer
- σy = standard deviation of stock Y
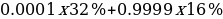
σ p = 16%
Thus, we now have the standard deviation of a portfolio and one is able to see that portfolio risk is sensitive to the proportions of funds devoted to each and the standard deviation of each.
The reduction of risk of a portfolio by blending into it a security whose risk is greater than that of any of the securities held initially suggests that deducing the riskiness of a portfolio simply by knowing the riskiness of individual securities is not possible. It is vital that we also know the interactive risk between securities (Harold, 1999).
The risk of the portfolio is reduced by playing off one set of variations against another. Finding two securities each of which tends to perform well whenever the other does poorly makes more certain a reasonable reruns for the portfolio as a whole, even if one of its components happens to be quite risky.
Risk premium
To allow a risk a business requires a premium that is above an alternative that is risk-free. The more uncertain one is about the future returns, the higher the risk and thus the greater the premium. The risk premium is included in the capital analysis of the business through discount rate. This rate is risk-adjusted because it allows for time and risk preferences. It represents the total of risk-free and risk premium and it reflects the investor’s attitude towards risk. Risk-adjusted discount rate is thus equal to risk free rate plus the risk premium. In Capital Asset Pricing Model, risk- premium is the difference between market rate of return & risk free rate multiplied by beta of the project. The risk-adjusted discount rate varies the discount rate depending on the degree of risk of investment, a higher rate being applied for riskier projects (Jordan and Miller, 2008).
Risk –adjusted discount rate is a method used to assess the relative attractive nature of projects. The capitalization rate of the project is pegged the nature of the cash flow of the system, the discount is directly proportional to the risks involved. This implies, the higher the risk the higher the discount. This is a procedure mostly taught in business school and is among the methods employed to comprehend project risks. The Capital Asset Pricing Model puts it in check. Capital Asset Pricing Model states that there is a linear relationship between the yield required on an asset and the asset’s contribution to portfolio risk. Therefore the greater the risks involved, the bigger the expected return on the investment.
Rate = risk free rate + (β× (expected return on the market- risk free rate) OR,
r = rf + (β × (rm – rf))
rm – rf is market risk premium. If the risks associated with a given investments are high then the risk-adjusted discount rates are also high while for less riskier businesses the discounts on premium capital are also lowered since the chances of risks occurring in the business are low. The risk free rate is 5%
r = rf + (β × (rm – rf))
12.5% = 5%+ β × (12.5%-5%)
12.5% = 5%+ β × (12.5%-5%)
7.5% β =7.5%
β =1.0
The Sharpe ratio of this investment will be
= Average return of portfolio – Risk free rate
Standard deviation of the portfolio
= 12.5% – 5% = 0.469
16
Adding pioneer to the market will improve the Sharpe ratio since is less than one.
Combining the Global mining and the market
The average return of this portfolio can be thought of as the weighted average return of each security in the portfolio; that is:
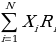
Where:
- Rp = expected return to portfolio
- Xi = proportion of total portfolio invested in security i
- Ri = expected return to security i
- N = total number of securities in portfolio
Therefore: Rp = (0.75%)(12.9%) + (99.25%)(12.5%) = 12.50%
Expected standard deviation
Then the standard deviation will be calculated as follows;
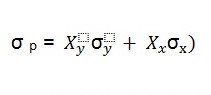
Where:
- σp = portfolio standard deviation
- Xx= percentage of total portfolio value in Pioneer
- Xy = percentage of total portfolio value in market
- σ x = standard deviation of pioneer
- σy = standard deviation of stock Y
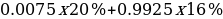
σ p = 16.03%
Thus, we now have the standard deviation of a portfolio and one is able to see that portfolio risk is sensitive to the proportions of funds devoted to each and the standard deviation of each.
Risk premium
Rate = risk free rate + (β× (expected return on the market- risk free rate) OR,
r = rf + (β × (rm – rf))
rm – rf is the market risk premium. If the risks associated with a given investments are high then the risk-adjusted discount rates are also high while for less riskier businesses the discounts on premium capital are also lowered since the chances of risks occurring in the business are low. The risk free rate is 5%
r = rf + (β × (rm – rf))
12.5% = 5%+ β × (12.5%-5%)
12.5% = 5%+ β × (12.5%-5%)
7.5% β =7.5%
β =1.0
The Sharpe ratio of this investment will be
= Average return of portfolio – Risk free rate
Standard deviation of the portfolio
= 12.5% – 5% = 0.468
16.03
Adding Global mining to the market will improve the Sharpe ratio since is less than one.
The Sharpe ratio measures the risk premiums of the portfolio where the risk premium is the excess return required by investors for the assumption of risk relative to the total amount of risk in the portfolio. It summarizes the risk and return of a portfolio in a single measure that categorizes the performance of the fund on a risk adjusted in a single measure that categorizes the performance of the fund on a risk adjusted basis. The larger the Sharpe ratio the better portfolio has performed.
Thus A ranked as the better portfolio because its index is higher, despite the fact that portfolio B had a higher return. The Sharpe ratio, which we are about to discuss have yielded very similar results in actual empirical tests.
Recommendation
I will recommend to the investor to buy shares of Global Mining because the standard deviation is lower but it has a higher return than Pioneer Gypsum. The investment in Pioneer Gypsum will generate almost the same rate of return but the standard deviation is high and they are likely to generate a negative return at one point in the portfolio life time.
Wildcat oil
The coal oil mine costs $ 5 million and the analysis is to recommend whether Jones should go ahead with the purchase of the mine. I have been assigned the task of recommending to the company as to whether it should go ahead with the acquisition of the coalmine.
Investment in the wildcat
In order to get the require rate of return for John and Marsha capital asset pricing model will be used as follows;
r = rf + (β × (rm – rf))
The risk free rate is provided in this case as 6%, beta is 0.8 and market premium 7%
r = 6%+ (0.8 × 7%)
r = 11.6%
You may determine the optimal sequence of decisions by “rolling back” the tree from the right-hand side. In other words, first appraise the most distant decisions; namely, the choice of whether or not to switch form regional to national distribution. To do so, determine the mean net present value for wildcat, given that production success and failure. The mean net present value is simply the net present values at the branch tips times the probabilities of occurrence.
They feel that there is a 30-70 chance that the production will be successful. Given an opportunity cost of capital of 11.6 percent. If not successful, they will discontinue the project and the cost of the field will be wasted. The firm is not obliged to enter full production, but it has the option to do so depending on the outcome of the tests. If there is some doubt as to whether the project will take off, expenditure on the pilot operation could help the firm to avoid a costly mistake.
You can probably now think of many other investments that take on added value because of the options they provide to expand in the future. In each of these you are paying out money today to give you the option to invest in real assets at some time in the future. Managers therefore often refer to such options as real option. These options do not show up in the assets that the company lists in its balance sheet, but investors are very aware of their existence. If a company has valuable real options that allow it to invest in profitable future projects, its market value will be higher than the value of its physical assets now in place. We consider the valuation of options.
The decision to terminate a project is usually taken by management, not by nature. Once the project is no longer profitable, the company will cut its losses and exercise its option to abandon the project (Peirson, Brown, Easton, Howard, Pinder and Sean 2000).
Using excel net present value calculator attached the net present value is 4,135,156 and internal rate of return of 28%. The production will continue for four years before starting to break-even.
If the output is known and shipping costs are fixed and all over sudden it changes to variable cost then the net present value will fluctuate from time to time depending on the cost production. in the case of operating leverage the company could be forced to adjust the expected cost of capital.
One must point out that the main difficulty associated with risky investments lies in obtaining cash flow estimates from people, not in the mathematical manipulation of the data obtained. People being what they are, biases invariably creep into the process. Sometimes the incentive compensation of managers is linked to the return on assets relative to some standard. If this standard is based on the expected return for investment projects, managers are likely to bias their estimates downward. In this way they are more likely to be able to exceed the standard. To ensure unbiased cash-flow forecasts, it is essential that the compensation of those doing the forecasting is divorced from subsequent performance(Whitman, 2000).
An individual may make unbiased cash-flow estimates for projects arising in that person’s area of responsibility. For some projects, these estimates will prove to be too high, whereas for others they will be too low. However, on average, the estimates are unbiased in the sense that over and underestimates cancel out. But not all projects are accepted. The forecasts are given to a higher level of management, which, in turn, makes the accept-reject decision. Acceptance, of course, depends on a project’s likely return relative to its risk. In this regard, there may be a tendency to select projects where costs are underestimated and revenues overestimated. Even though a person’s overall estimates are unbiased, this tendency will result in those selected being biased. If this happens, actual returns on accepted investment projects will be lower on average than their projected returns. Decision tree approaches have been advanced for adjusting for this type of bias (Sharpe, 2000).
In the adjustment for biases, one problem faced in any organization is over adjustment. Sam makes a forecast that he regards as unbiased and sends it up through the chain of command for final project approval. Also, there is the problem of accountability. Because capital investment projects involve returns over many years, it is difficult to go back to the person who made the forecast with the actual results. That person often has been transferred or has left the company. While the best approach to correcting biases may be to present a forecaster with the actual results for a number of projects and to compare these results with the forecasts, this often is not possible for long-lived projects (Brealey, Myers and Marcus, 2007).
In addition to the biases described, others also are possible. Although the focus is on the quantitative organization of data, we must be mindful of the fact that the accuracy of the final results depends heavily on behavioural considerations. Every effort must be made to provide an environment conductive to the unbiased forecasting of project cash flows.
Conclusion
The risk in this venture is very high as the net present value shows that the return on capital is higher than the cost of capital. The probability of occurrence of negative outcomes (loss), in this venture is very high.
Reference List
Brealey, R., Myers, S., & Marcus A., 2007. Fundamentals of corporate finance. Sydney: McGraw-Hill.
Fischer, D., & Jordan, R., 2007. Security Analysis and Portfolio Management. New Delhi: Prentice-Hall of India Private Limited.
Gordon, A., Jeffrey, B., & Sharpe, W., 2000. Fundamentals of Investments. New York: Prentice-Hall.
Harold, B., 1999. Corporate financial strategy and decision making to increase shareholder value. Pennsylvania: Frank J. Fabozi Associates.
Jordan, B., & Miller, T., 2008. Fundamentals of Investments Valuation and Management. Boston: McGraw-Hill Irwin.
Peirson, G., Brown, R., Easton, S., Howard, P. & Pinder, S., 2000. Business finance. The McGraw-Hill Companies, Inc, Sydney.
Sharpe, W., 2000. Portfolio Theory and Capital Markets. New York: McGraw-Hill.
Whitman, M., 2000. Value Investing: A Balanced Approach. New York: John Wiley and Sons.