The classical time-inconsistency problem analysed by Kydland and Prescott (1977) and Barro and Gordon (1983), and followed by a large literature, is based on the following model: y = γ (π – π ) + u , where yt is the log of output (or employment), measured as a deviation from potential output, πt is the rate of inflation at period t, and πt e is the rate of inflation at period t expected by the private sector at period t-1. ut is supply shock, which we assume is white noise, and γ is a positive constant. Equation 1 is a “Lucas supply curve”, where inflation surprises reduce the real wage and stimulate output. The private sector is assumed to have rational expectations, so that: πt= E t-1πt, where E t-1 is the mathematical expectations operator based on information available at period t-1. The crucial assumption is that private sector form their expectations (set their wages and prices) before inflation is realised. The policymaker has preferences over inflation and output, which are represented by the following loss function:
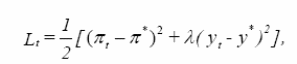
where λ > 0 and y* > 0. The policymaker is assumed to minimise the sum of current and discounted future losses. However, since the model is static, we can consider only minimising the period loss given by 3. Note that the policymaker’s output target, y*, is higher than potential output, which is normalized to zero. The reason for having an over-ambitious output target might be a labour market distortion that gives too high equilibrium unemployment. The socially optimal rate of inflation is for simplicity assumed to be zero, although a positive rate would not affect the results. The monetary policymaker is assumed to control the rate of inflation πt. The discretionary solution can be found by minimizing Lt with respect to πt and subject to 1 and 2. This results in the following firsts order condition: (pt – p*) 2 + g l(yt – y*) = 0
This gives the following solutions for inflation and output:
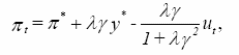
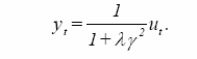
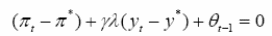
To show that the discretionary equilibrium, given by 5 and 6, is sub-optimal, we first need to find the optimal policy rule under commitment. This can be found by minimizing Lt with respect to both πt and πte, which gives the following first-order conditions

where θ t-1 is the Lagrange multiplier corresponding to 2.
These give the following outcome:

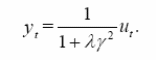
We see that the only difference between the discretionary equilibrium and the optimal commitment equilibrium is that the former gives too high average inflation. The inflationary bias is given by λγy*. Note that the policy responses to shocks are identical in the two cases. Thus, although being too expansionary on average, a discretionary monetary policy provides optimal stabilization. Then, a discretionary policy gives rise too an output stabilisation bias.
The reason for the time-inconsistency problem in this model is that private expectations are formed before the government sets the inflation rate. If private agents set wages under the assumption that inflation will be π*, the government has an incentive to increase inflation in order to stimulate output. However, rational agents recognise the government’s incentives, so that they expect inflation to be higher than π*. Inflation expectations become just high enough so that the marginal cost of inflation is equal to the marginal gain from higher output, such that the government has no incentive to surprise.
If the government were able to commit to in inflation rule given by 9, the problem of time-inconsistency would be solved and reduced to an “engineering” problem of finding the optimal rule. However, state-contingent rules like 6 are considered difficult to implement in practice, partly because of the difficulties in legislating various contingencies. Non-state-contingent rules may therefore be the only feasible type of rules. However, although removing the inflationary bias, commitment to non-state-contingent rules results, in general, in sub-optimal stabilisation. The difference between discretion and rules may then be viewed as a difference between optimal stabilization and price stability. The distinction is, however, a continuum rather than a dichotomy. As formulated by Lohmann (1992), there is a trade-off between credibility and flexibility. Much of the literature has considered solutions that provide an appropriate balance between credibility and flexibility.
The proposed solutions to the classical time inconsistency model can be grouped into reputational solutions, delegation solutions and contract solutions.
The analysis above rests on an assumption that the game between the policy maker and the private sector is a one-shot game. The argument against discretion may be weaker if a dynamic game is considered. The policy maker may then be willing to sacrifice some short-term gains by investing in reputation today and harvest the gains in the future.
Two alternative ways of modelling “reputation” are applied in the literature. One approach is to model reputation as the use of a “trigger strategy”, as applied by e.g. Barro and Gordon (1983b). The private sector sets low wages (or inflation expectations) as long as the policy maker sets low inflation, but “punishes” the policy maker by setting high wages the subsequent period if the policy maker sets high inflation. The “punishment” strategy of the private sector creates incentives for the policy maker to preserve a “reputation” of setting low inflation. In a finite horizon game, there exists equilibrium where the policy maker sets low inflation until the last period, in which he sets high inflation. A condition for this equilibrium to be realized is that the policy maker does not discount the future too heavily.
A second approach to modelling reputation, as applied by e.g. Backus and Driffil (1985), is to assume that the private sector is uncertain about the true preferences of the policy maker. The private sector may expect the policy maker to be either “tough” on inflation or “weak”. By Bayesian updating based on the monetary policy outcome, the private sector attaches a subjective probability to the policy maker being “tough”. This creates an incentive for a “weak” policy maker to build a (false) reputation of being “tough” by setting low inflation.
A problem with the reputational solutions to the time-inconsistency problem is that they quickly become very complex and give rise to multiple equilibria, which gives the approach limited predictive power. Multiple equilibria also raise the problem of how the government and private agents can coordinate on one equilibrium. Moreover, although improving the one-shot discretionary equilibrium, reputational solutions do not implement the second-best equilibrium, i.e. the outcome of the optimal rule under commitment.
As a compromise between credibility and flexibility, Rogoff (1985) suggested that the government should appoint a “conservative” central banker, that is, a central banker with the following preferences:
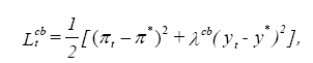
where λcb is the central banker’s subjective weight attached to output stability, λcb < λ, that is, the central banker’s weight on output is smaller than the society’s weight. The intuition can be explained as follows: Reducing bc marginally from the point bc = b gives a first-order (“large”) gain in terms of lower average inflation and a second order (“small”) loss in terms of reduced output stability. Increasing bc marginally from the point bc = 0 gives a first-order gain in terms of increased output stability and a second-order loss in terms of higher average inflation. Thus, the optimal bc must lie between zero and b.
Lohmann (1992) showed that the outcome can be further improved if the government complements the appointment of a “conservative” central banker with an option to override the central banker at a fixed cost. The intuition is that delegating the conduct of monetary policy to a “conservative” central banker is costly for the society if large shocks occur. Thus, the government will pay the price of overriding if large shocks occur and set monetary policy at its own discretion. The central banker dislikes being overridden and pays attention to the government’s preferences when responding to large shocks. In equilibrium, the central banker is never overridden.
Although improving the discretionary equilibrium, the reputational and delegation solutions to the time-inconsistency problem do not provide the second-best equilibrium. In order to achieve the second-best equilibrium, various contracting solutions have been considered. The relationship between the government and the central banker may be considered a “principal-agent” problem. The government (the “principal”) delegates the conduct of monetary policy to a central banker (the “agent”) with certain incentives. The government can affect the incentives of the central banker by specifying a contract between the central banker and the government. Walsh (1995) showed that the second best equilibrium can be achieved by offering a linear inflation contract to a central banker with the same preferences over inflation and output as the government. The central banker’s loss function may then be written as:
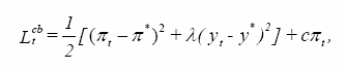
where the contract enters as an additive term in the loss function. It can easily be shown that the optimal policy is achieved if c = λγy*, i.e. the marginal penalty for generating inflation is equal to the inflationary bias under a discretionary policy.
Central bankers tend to reject the classical time-inconsistency problem that hinges on an over-ambitious output or employment target. One reason for this is that central banks are much more independent from political authorities today than they used to be when this literature was in focus, and there is a general consensus that they central banks should stabilise output at its potential rather than the level desired by politicians.
The literature may, however, contribute to explaining why inflation was high during the 1970s and 1980s, and how it could be fought. For example, Paul Volcker, who was Chairman of the Federal Reserve in the period 1979 – 1987, was considered a “conservative” central banker in Rogoff’s (1985) meaning. By focussing almost solely on inflation, he succeeded in bringing inflation under control. Although it is difficult to make contra-factual analyses, it seems fair to say that the delegation of monetary policy to a “conservative” chairman as Volcker helped to end “the great inflation”.
Norway pursued another strategy to achieve price stability than appointing a “conservative” central bank governor. After a long period of high inflation and frequent devaluations, there was a political consensus after the devaluation in 1986 that this devaluation should be the last one. The economic policy after 1986 could therefore be interpreted as commitment to a fixed exchange rate. The commitment was not without costs, however. After the German unification, the interest rate in ERM increased considerably, and the timing was bad for Norway, which experienced large imbalances after the credit market liberalisation in mid 80-s. The result was record high unemployment and a banking crisis.
To conclude we may say that in some situations policymakers may want to announce in advance the policy they will follow to influence the expectations of private decisionmakers. But later, after the private decisionmakers have acted on the basis of their expectations, these policymakers may be tempted to renege on their announcement. Understanding that policymakers may be inconsistent over time, private decisionmakers are led to distrust policy announcements. In this situation, to make their announcements credible, policymakers may want to make a commitment to a fixed policy rule. Consider the dilemma of a Federal Reserve that cares about both inflation and unemployment. According to the Phillips curve, the tradeoff between inflation and unemployment depends on expected inflation. The Fed would prefer everyone to expect low inflation so that it will face a favorable tradeoff. To reduce expected inflation, the Fed might announce that low inflation is the paramount goal of monetary policy. But an announcement of a policy of low inflation is by itself not credible. Once households and firms have formed their expectations of inflation and set wages and prices accordingly, the Fed has an incentive to renege on its announcement and implement expansionary monetary policy to reduce unemployment. People understand the Fed’s incentive to renege and therefore do not believe the announcement in the first place. Just as a president facing a hostage crisis is sorely tempted to negotiate their release, a Federal Reserve with discretion is sorely tempted to inflate in order to reduce unemployment. And just as terrorists discount announced policies of never negotiating, households and firms discount announced policies of low inflation. The surprising outcome of this analysis is that policymakers can sometimes better achieve their goals by having their discretion taken away from them. In the case of monetary policy, there will be lower inflation without higher unemployment if the Fed is committed to a policy of zero inflation.
Reference
Walsh, Carl, “Optimal Contracts for Independent Central Bankers”, American Economic Review 85, 1995:150-167.
Walsh, Carl. Monetary Theory and Policy. MIT Press 2003: 363 -428.